Circles: Slope of the
Line Segment from Point to Center vs. Slope of the Tangent Line
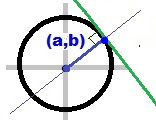
Consider a circle with point (a,b) on the circle. Let’s compare the slopes of two lines:
(I) The line connecting the center of the circle and the
point (a,b), and
(II) The slope of the tangent line containing point
(a,b). The tangent line is a line that
touches the circle at point (a,b).
Case 1: The center is
(0, 0) (at the origin)
The equation of the circle is x^2 + y^2 = r^2, where r is
the radius.
Since (a, b) is on the circle, then a^2 + b^2 = r^2.
(I) Slope of the line connecting the center (0,0) and point
(a,b). Then the slope (labeled s1) is:
s1 = (b – 0)/(a – 0) = b/a
(II) Slope of the tangent line connecting (a,b). The slope at point (a, b) would also be the
slope of the tangent line.
Solving for y,
x^2 + y^2 = r^2
y^2 = r^2 – x^2
y = √(r^2 – x^2)
The derivative is:
dy/dx = 1/2 * (-2 * x) * (r^2 – x^2)^(-1/2)
dy/dx = -x / √(r^2 – x^2)
At point, (a, b), the slope (labeled s2) is:
s2 = -a / √(r^2 – a^2)
Since a^2 + b^2 = r^2,
s2 = -a / √(a^2 + b^2 – a^2)
s2 = -a / √(b^2)
s2 = -a/b
Note: Since s1 = b/a and s2 = -a/b, then s2 = -1/s1.
Case 2: The is center
is at point (m,n) with point (a,b) on the circle.
The equation of the circle becomes (x – m)^2 + (y – n)^2 =
r^2.
(I) Slope of the line
connecting the center (m, n) and point (a,b).
Then the slope (labeled s1) is:
s1 = (b –n)/(a – m)
(II) Slope of the tangent line connecting (a,b).
Solving for y leads to:
(x – m)^2 + (y – n)^2 = r^2
(y – n)^2 = r^2 – (x -,m)^2
y = √(r^2 – (x – m)^2) + n
Taking the derivative:
dy/dx = -(x – m)/√(r^2 – (x-m)^2)
With the point (a,b):
s2 = -(a – m)/√(r^2 – (a – m)^2)
s2 = -(a – m)/√((a – m)^2 + (b – n)^2 – (a – m)^2)
s2 = -(a – m)/(b – n)
Since s1 = (b – n)/(a – m) and s2 = -(a – m)/(b – n), them
s2 = -1/s1
Conclusion: On a circle
with center (m,n), with the point (a,b) on the circle, the slope of the tangent
line through the point is –(a – m)/(b – n).
Eddie
This blog is property
of Edward Shore, 2016.